Music Philosophy
A great battle about music from history (Part 4)
This is part 4 in this series. You can read the first three parts here:
Part 1
Part 2
Part 3
My experience in tuning pianos is limited to a college class I took from a local college many years ago. I have all the tools to tune a piano but would never think of touching my own piano. I know the result would be disastrous. Tuning is a very complicated business, mostly because of a concept we call temperament.
In music, temperament refers to moving pitches around slightly to get a certain result. Not surprisingly, there are numerous ways to tune and debates have raged for centuries over how tuning should take place.
We have talked about how the original ideas of tuning came from the ancient Greeks who saw natural laws of numbers in sound and decided that we should tune according to those laws. The church jumped on the bandwagon, and for the most part, tuning was done the Greek way until somewhere in the area of 1500 AD.
Here is the essence of the Greek tuning:
- Octaves should be tuned so the ratio between the top note and bottom note is 2:1. In ancient times, that might be done by measuring the strings of each note. Today, we think in terms of frequency. To this day, if you have an A at 440 Hz, the A above it is tuned to 880 Hz.
- Perfect fifths should be tuned with ratio of 3:2.
- Perfect fourths should be tuned to 4:3.
- No other intervals were really considered. All other notes were tuned by using a combination of these intervals. It is sort of complicated to explain, but if you think about this a minute, you will see how it worked. You can indeed start on any note on the piano and reach every other note using a combination of fourths, fifths and octaves.
Sounds great doesn’t it? But there is a big problem and it has to do with math. The math just does not add up.
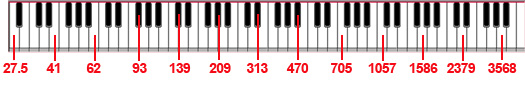
The one ratio that is pretty much imperative is indeed that 2:1 ratio between octaves. That really is the basis for everything. Here is a keyboard with each A marked in the Hz frequency that we need to achieve a 2:1 ratio between all the A’s. Notice that the frequency of each note is simply multiplied by 2.

Now stay with me here. Pythagoras had a simple theory that he wanted to test one day. He knew that if you started on a note and then moved up perfect fifths, you would eventually get back to the same note some number of octaves later. This concept is correct. We call it the circle of fifths. If you start on any note and move up or down 12 fifths, you will play all 12 notes found in a chromatic scale and eventually get back to the same note 7 octaves later.
Or, look at it this way. Start on that low A and start counting fifths. You go to E, B, F#, C#, G#, D#, Bb, F, C, G, D, and back to A.
So Pythagoras started measuring using his 3:2 ratio. He did indeed eventually get back to the same note 7 octaves higher, but the note was out of tune. Here is what happened.
Note that the frequency of the top A is different from the frequency achieved by using the 2:1 octave ratio from the first example.
I am not going to bore you with the explanation, mainly because I do not quite understand it myself. It has to do with the nature of prime numbers. Concepts like prime numbers were not really understood at that time. By the way, if you tried to do this with perfect fourths instead of perfect fifths, you would run into exactly the same problem.
Now, here was the dilemma. Most musicians would probably prefer the sound of a perfect fifth or a perfect fourth. But if you tune instruments using perfect intervals, problems arise. Because those perfect fifths are just a little bit wider than they should be, they make other intervals narrower. That leads to some intervals that just do not sound very good. These intervals are referred to as “wolf intervals”, and musicians that played those tunings had to learn to avoid them.
As the debate raged, many different solutions were proposed. There are historical references to organs where the black keys were divided into two or even three pieces (each with a slightly different pitch) to account for various scenarios.
Eventually, equal temperament was widely accepted and is used to this day. Equal temperament is a pragmatic but imperfect solution. The concept of truly perfect fifths and fourths is surrendered so that no “wolf intervals” will exist. The octave is essentially cut into 12 pieces where each note is exactly the same difference apart.
Today, equal temperament seems like a logical and even obvious solution, but at the time, it was not. Remember that equal temperament was considered an affront to the laws of the church and nature. After all, who were we to change God’s 3:2 ratio in a perfect fifth?
And to be honest, to this day, you will find musicians who don’t like equal temperament. In fact, there are professional musicians that specialize in playing with old tunings. So, the debate rages on.
And so will this series…
